Saturday, June 1, 2013

Differentiation Rules - Finding the Derivative of a Difference of Functions

This post continues along in my series on calculus differentiation rules, this time talking about how to find the derivative of a difference of functions. I hope you read my last post, which applied to sums of functions, because it is nearly the same situation when you are subtracting. I'm not going to go into the same level as detail as I did there, so I highly recommend you go back and give that a read, and then come back here to see how the same kind of thing applies.

Recall the setup for these rules. Let f(x) and g(x) be two separate functions (anything you want), and let's also say that the sum of these functions, that is, f(x) + g(x), is equal to F(x). Similarly, let's say that the difference of these functions, that is, f(x) - g(x), is represented by G(x).

To find the derivative of a difference of functions, you simply determine the derivatives of the component functions and subtract them accordingly to get G'(x).

Therefore: the derivative of a difference of functions is equal to the difference of those functions' derivatives.

Compare this to the differentiation rule for adding that I covered last time. You can see how it's the same kind of thing, with no extra manipulations or reorganizations or tricks. If you can add, then you can subtract.

I'm not going to provide any examples for this rule, unless anyone leaves me a comment below to specifically request some. I think that if you can work through the example for adding, you will have a good grasp of how to perform both the addition and subtraction differentiation rules.

Thanks for checking out my latest post, even though this is one of my shorter ones. I always love getting feedback, and Facebook Likes and +1's are very much appreciated!

Thursday, May 9, 2013

Differentiation Rules - Finding the Derivative of a Sum of Functions

Welcome back to my introductory calculus series on differentiation formulas. For those who are playing along at home, I have explained several rules so far and am going to add another one today. If you've missed those posts, then I highly encourage you to go back and take a look at them to familiarize yourself with these basic concepts. (So far: here, here, and here. Or just check my table of contents to find more!) Today, I am going to talk about finding the derivative of a sum of functions.

Consider that you have two functions. They can be whatever you want, it really doesn't matter. While I explain this concept, let's just call these functions f(x) and g(x). Now, suppose that you want to add these functions together, and you come up with a third function, let's call it F(x). Sounds complicated? It doesn't have to be. It's actually quite simple. But what about if we want to find the derivative F'(x) - how do we do that?? That's easy too. You just need to understand this property of derivatives.

All you need to do is find the derivatives of the "smaller" functions, f'(x) and g'(x), and then add those together to get F'(x)!

Put simply, the derivative of a sum of functions is equal to the sum of those functions' derivatives.

In other words: F'(x) = f'(x) + g'(x).

Let's try an example, and put some numbers to this thing so that you can see it's not that crazy.

Keep in mind that when I talk about functions here, these could be anything: x², or (x-2)^5/16. Simple, or more complicated. The same rules apply. For now, let's keep it simple.

Suppose that f(x) = x² and g(x) = x³. What is the sum of these, F(x)?

Well this is as easy as it gets. What is the sum of x² + x³?
Your new function F(x) is just that: F(x) =x²+ x³.

So then, differentiate F(x) to find its derivative, F'(x).

By the rule that I explained above, all you have to do is find the derivative of the individual pieces of this sum, and then add those! So, by the power rule, we have:

F'(x) = 2x + 3x²

That's it!

Obviously this can get a heck of a lot more complicated, but the principle remains the same. What if you want to find the derivative of some G(x) = x⁴+ x² + 4x^2/3. Think of this as a sum of three smaller functions, and you can see how this rule applies here. You don't need to necessarily always have two distinctly different functions to apply this. Think back to what a function is - they can have many terms to them. (They just have to pass the vertical line test!)

My point in all of this rambling is that to find derivatives of functions that have multiple terms in them added together (like G(x) above, or F(x) before that), all you need to do is find the derivatives of the individual terms, and add them together. It really is quite straightforward, and I hope that I have made helped to make this clear. Once you get in the habit of applying this rule, you will do it automatically.

Please let me know if this has helped you, or should be clearer in some way. I appreciate any feedback you would like to leave! Also, please do me a favour and click on the Like or +1 buttons if this post helped you in any way.

Wednesday, May 8, 2013

Happy Fibonacci Day!

I know this may be a little late in the day, but I just realized that today's date is actually a Fibonacci sequence! That's right, today's date is May 8, 2013, or written another way, 5/8/13!

For those who don't know, the famous Fibonacci sequence is starts off with the numbers 0, 1, and then continues by adding numbers that are equal to the sum of its preceding two numbers. So, the classical sequence is 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55.... you can keep going on and on if you wish.

Today's date, when written as I have above, forms a section of this sequence. If you would rather write your date in the format of month/day/year, then you can expect another Fibonacci day on August 5, 2013. Unless I'm mistaken, there won't be another one of these nice alignments of date numbers until August 13, 2021! So, I'm sorry I didn't recognize this earlier in the day to share with everyone!

Saturday, May 4, 2013

Differentiation Rules - Finding the Derivative of a Constant Times a Function

In this post I'm going to explain another one of the differentiation rules for working with derivatives. This time, I will show you how to find the derivative of a constant times a function.

In case you have missed them, I am creating a series of posts that explain some basic concepts in differential calculus. So far, in my first lesson I explained how to find the derivative of a constant function, and then I followed that with a post about the power rule for derivatives. So far, these are some of the basic rules for calculating derivatives, and it is a good idea to become very familiar with them so that you can apply them all as required on more elaborate problems later on.

This derivatives rule is a very simple one, but that doesn't make it any less important. Consider that you have any function f(x), and it is multiplied by a constant. Assuming that f'(x) in fact exists, f'(x) times a constant is equal to the constant times this derivative. That may sound a little wordy, so maybe this equation will be a little clearer:

So, all you really need to be concerned with is calculating the derivative f'(x). Then, multiplying by the constant is a simple calculation that you can add at the end.

If you're curious about what this rule means, here is one way of looking at this. If you have an equation such as y = f(x), if you consider multiplying this function by a constant, what you are doing is essentially stretching the graph vertically. So, comparatively, y = 2f(x) is a graph that is twice the height. Therefore, if you then consider the slope along this function, since you have doubled the rise but not the run (the slope calculation), you have a doubled slope at every point. And since the derivative of a function represents the slope of the line at a point, you can then see how this rule all comes together. Basically, if you stretch the function by a constant factor, you can simply multiply the slope (the derivative) by this factor as well.

As I said, there isn't much to remember about this particular derivative rule, but it is very important to know. It will often need to be considered in addition to other rules that I have/will outline in this series. One example would be to calculate the derivative of something like 4x³. In this case, you'd need to draw upon this rule, as well as the power rule from my last post. There are a few other basic differentiation rules like this one that I will cover in my next posts. Learn all of these rules well, and you'll have no problem differentiating complicated functions!

One final comment - if you thought this post was helpful in any way, then please do me a favour and click on the Like and +1 buttons found on this page! Thanks for your support and for visiting. Be sure to come back if you need help with any other maths concepts.

Tuesday, April 30, 2013

Differentiation Rules - The Power Rule

Welcome to my second post of my series on differentiation formulas. So far in my recent posts, I have explored in depth all about the concept of derivatives and using differentiation to find them, and then I started this current series with an easy theorem to remember for finding the derivative of a constant function. This follow-up post will now explain to you probably one of the most used methods for finding derivatives: The Power Rule for Differentiation.

First, allow me to present this rule to you in all it's nasty-looking glory:

The only condition that this rule has is that n must be a positive integer. OK, maybe it's not as frightening as I would have made it out to be... but it still doesn't look very friendly. However, when you start doing questions with it, you will understand the easy workflow process and find that it actually is very simple to remember and use!

Let's try a few. Trust me, you will see the pattern and rhythm right away!

Find the derivative of f(x) = x³.

According to our rule, the n value here is 3, so then we have:

f'(x) = 3x^3-1 = 3x².

That's all there is to it!

Next, differentiate the function f(x) = x⁵⁰⁰.

This one seems a bit tougher, but you do the exact same thing. Check it out:

f'(x) = 500x^500-1 = 500x⁴⁹⁹

Easy, right?

Here's a simple image that can help you to remember what you have to do. Like I said, once you start doing these, it is very hard to mess up.

Here's a better way to remember this, which may be even easier to think of but harder to draw: imagine that the exponent is a pile of sequential number cards (e.g. 1, 2, 3, 4, 5...) with the high card on top. When you have to find the derivative, take the top card and place it in front of the x, leaving the card beneath it (which is 1 number smaller) visible. It's easier to actually do this than to draw it, but it's a great way to remember this formula because it is so easy to visualize!

For fun (!), you can see the proof of this by using the Binomial Theorem. I won't go into that much detail here, but in a nutshell, if you start with the definition of a derivative and let f(x) = xⁿ, you can expand the (x + h)ⁿ term with the Binomial Theorem. Since the definition requires you to find the limit as h approaches zero, this causes all but the first term of the expansion to equal zero, leaving you with the above result.

So, now you know one of the most common differentiation rules. In my next posts, I'll show you how to apply this rule to your terms when you have a constant value in front of the x. It's as easy as to do as this one! Thanks for reading, and make sure you click the Like and +1 buttons on this page!

Tuesday, April 23, 2013

Differentiation Rules - Derivative of a Constant Function

For those of you just tuning in, my last post was a mega-post about derivatives and an introduction to differential calculus. If you need some help getting started with understanding how to find derivatives, I highly recommend giving that a read. One impression you may have of this concept is that it requires a lot of work - lots of lengthy formulas and limit calculations. While you could certainly use those methods for all of your differentiating questions, you would be wasting your time! In my never-ending quest to show you mathematics made easy, today I am going to start a series of posts about differentiation formulas - essentially, shortcuts through a lot of the repetitive and lengthy work needed if you were to explicitly use the definition of a derivative! Once you learn some of these tricks, you will fly through your calculus homework!

(To be honest, these aren't so much tricks as they are actual mathematical theorems and rules.)

The first rule should be simple to understand, if you think about it. Take any constant function, f(x) = c. The derivative of this, that is, f'(x), will always be zero.

It's an easy one to remember, and the explanation is easy to visualize as well. If you have a graph of f(x) = c, you basically have a horizontal line that never varies. For every value of x on your curve, f(x) is the same, constant value. So, it has no slope - its slope is zero. Furthermore, if you refer back to my graphical explanation of derivatives in my previous post, you will see that the derivative f'(x) is equal to the slope of f(x). So, if we have a line that has no slope here, we can see how this rule comes together. For fun, you can practice your limit notation and long-hand derivative calculations to prove that this is the case. Refer back again to my last post and use the definition of a derivative. Hint: in your calculation, f(x) = c, and f(x + h) = c.

This is one of the easiest differentiation formulas (if you can call it that) that you are going to encounter, so memorize this one, and get ready for something a little bit more challenging in my next post: the Power Rule.

Please remember to click the Like and +1 buttons on this page if it was helpful! I really appreciate it!

Thursday, April 11, 2013

Derivatives and an Introduction to Differential Calculus

One of the main concepts studied in the field of differential calculus is based on the notion of change - specifically, how one quantity changes compared to another. Perhaps a more succinct version of this physical definition would be "rate of change." Alternately, a geometric definition could simply be the slope of a curve at a particular point. The underlying key to this branch of mathematics is the concept of the derivative. In this post, I will introduce various aspects of the derivative - please click the Facebook Like button if this is helpful for you!

First, let's consider the derivative in terms of rate of change. And to do that, let's talk about velocity. We know that velocity is equal to distance per unit time. However, that is a very general description of it. If you drive from your home to the grocery store on the other side of town, you can do the math by dividing your total distance travelled by the time it took you to get there, but what does this number tell you? It actually tells you the average velocity of your trip. Think about it. You had to stop for red lights, stop signs, pedestrians. Maybe you sped up to pass a slow driver. Don't forget about the actual acceleration of your car from a standstill, and then the deceleration whenever you needed to stop. All of this factors into the calculation of your average velocity, which is simply how far you go in a measured amount of time.

Now, let's consider how to calculate the average velocity of your car between your home and the first stop sign, while on your way to the grocery store. You no longer have several stops to deal with. You get in your car, accelerate, then as you approach the stop sign, you decelerate to a stop. Your average velocity is calculated from a much shorter interval, and will have much less variation to it. So, your average velocity will be more representative of your actual velocity at any given time.

To extend this demonstration even further, let's consider the small portion of your trip that is measured between two street lights 10 meters apart that you pass while you are in full motion. That is, let's assume that we want to measure the velocity without having to calculate stop signs, etc. Our time interval for the measurement is much smaller, and calculating the velocity by dividing the distance by the time it takes to go from one light to the other is even more representative of your velocity at any point between them.

What I am trying to demonstrate is the concept of instantaneous velocity. If you shrink down your time interval of measurement infinitesimally, the two time points approach each other at a single point, and so the average velocity between the two super close points approaches the instantaneous velocity of the single point.

Graphically, this is essentially the same thing you do when you calculate the slope of a tangent line to a curve. You pick two lines on the curve and calculate the slope of the line between them, and then you use limits to make the points get closer and closer together until they are almost the same, and the slope of the line connecting those two infinitely close points is the tangent. (Check out my previous post about using limits to find tangents if you'd like a refresher of this topic: http://sk19math.blogspot.ca/2012/06/using-limits-to-find-tangents.html)

Because this type of limit occurs so frequently in maths, science, and engineering, it is given the special name of "derivative," and you calculate derivatives through the process of "differentiation." So, one interpretation of the derivative is an expression of the instantaneous rate of change (velocity) at a particular point on the curve - a large derivative corresponds to a high rate of change (a steep curve), and conversely a small derivative corresponds to a low rate of change (a relatively flat curve). As a specific example, if you actually have a graph of position (displacement) of an object vs. time, the derivative of the curve at any time point represents the velocity of that object at that specific time. This may take a little practice to become comfortable with the concept, but suffice it to say at this point that learning how to use derivatives is incredibly important to be able to work out more complex concepts relatively easily.

Let's look at this now in the more formal terms of mathematical symbols and equations. Consider any curve y = f(x).

Now, let us identify the point P on the curve f(x) for when x = a. That is to say, the point (a, f(a)).

Now, let's go a step further, and identify a point Q that is h units away from a on the x-axis. If it is h units away from a, we can call it "a + h". (If this is confusing, think about it with numbers instead. Start at, say, x = 3 (instead of a). Now we want to know what is going on 5 units (instead of h) away from x = 3. In other words, we have 3, and we have 3 + 5.) As such, we can therefore identify a point Q ((a + h), f(a + h)).

Now that we have two arbitrary points, let's determine the slope of the straight line that would connect the two. We can use the same slope formula that we always use, slope = rise/run, but substitute in our variables that we identified above. So, we have:

Now, imagine that the distance h between the two points is getting smaller and smaller. Or in other words, consider the case of when h approaches 0. By doing this, we calculate the slope of the line connecting two infinitesimally close points - which means that we are actually approaching the slope of the tangent line to the curve at point a. In this case, we would express this slope as a limit in the following way, which actually corresponds to the definition of the derivative of a function f at a number a. The derivative is given the special symbol f'(x), and we say "f prime x", and we express it like this:

Another way of expressing this can be found if we recognize that a + h is really just any x value. So, we can say x = a + h (and by extension, h = x - a), and modify the above derivative definition accordingly:

With this modified equation, it actually becomes a matter of arithmetic to determine the slope at a point. Here is an example of a kind of question that you will see:

"Find the derivative (or, determine the slope of the tangent) of the function f(x) = x² - 4 at a number a."

To do this, write the provided equation into the definition, and reduce until you have an answer. Notice below how I combine terms and recognize the identity of a difference of squares.

What this final result tells you is that for our curve, f(x) = x² - 4, at any number a along it, the slope of the tangent (AKA, the derivative at that point) is equal to the term 2a. Graph it out and try with several values to convince yourself that it's true! Consider when x = 5. You can determine from the original equation that we have the point (5, 21). At this point on the curve, the slope of the tangent equals 2 x 5 = 10.

Going back to the definition of the derivative that I gave above, you can also apply the concept of point-slope form to it to get a different way of seeing it. Letting y = f(x), you can rearrange the definition as follows, by simple reorganization of the terms:

Here's a more visual exercise that you may soon encounter.

"If you are provided a graph of a function f(x) - not necessarily the equation - sketch out what the graph of the derivative f'(x) would look like."

When you actually have the numbers and equation, this becomes much easier... assuming you know how to easily recognize derivatives from the original equations. However, if provided ONLY the picture of the curve, this becomes a bit more abstract, but not really that challenging. It DOES require you to understand the concept of derivatives and rate of change though. Here is why. Take some random curve that you can draw. Any curve will do for this exercise:

The key is rate of change. We have seen that slope is equal to rate of change, so we want to pay particular attention to the slope at several points. And the easiest points to notice are those where the slope is equal to 0. These are all the peaks and valleys of the curve. What I have done next is highlight with red bars all of the zero-slopes:

Now, to proceed with sketching the graph of the derivative f'(x) vs x, you can start by plotting the points where f'(x) is equal to zero. From there you can then go on to say where the curve of f(x) has a positive, increasing slope, and then sketch that into your f'(x) graph accordingly. Similarly, decreasing slopes on the f(x) curve will be negative values on the f'(x) curve. For the sake of this exercise, don't worry so much about how high or low the slopes are. Just focus on whether they are positive or negative at the various parts of the graph. I have gone ahead and plotted out the actual curve of the derivative below in green, alongside the original curve of f(x). You can see that the f'(x) curve crosses zero wherever the curve for f(x) has peaks or valleys, and the steeper the f(x) curve, the more extreme the f'(x) curve at that same point.

Of course, having a mathematical definition wouldn't be any fun if there were no conditions or rules associated with it - and the definition of derivatives is no exception. One such rule states that a function is differentiable at a point a if the derivative f'(a) exists. Seems intuitive enough. If a derivative at a point exists, then the base function is differentiable at that point. Probably one of those rules that doesn't really even need to be said. :)

I'm not going to graph this one out, but it is for you to think on. Consider the case of f(x) = |x|. Where is it differentiable?

If you consider the derivative of the left hand side, it equals -1. The f'(x) on the right hand side is 1. This function then is obviously differentiable when x < 0, and when x > 0. But what about when x = 0? In this case, since the right hand limit approaches 1 as x approaches 0 from the right, and the left hand limit approaches -1 as x approaches 0 from the left, one must conclude that f'(0) does not exist because both of the one-sided limits approach different numbers.

An extension of this example actually describes a second rule for limits: if f'(a) exists, then the function f(x) is continuous as a. Recall that continuity of a curve is based on the notion that as you approach a point from both the left and the right, the limit of each side approaches the same value. In the example above, approaching 0 from either side resulted in different limits, and hence the graph is not continuous at 0.

Keep this in mind as you see various graphs of functions. Curves that have a sharp point will not be differentiable at the point, for the reason given above. Similarly, discontinuous curves (i.e. curves with gaps in them) will not have a derivative at the break point either because the one-sided limits do not agree. If f(x) is not continuous as point a, then f'(a) does not exist. A third condition to watch out for is where a graph has a vertical tangent line, in which case the slope is infinite.

Now, I'm going to wrap up this mammoth of a maths post with something a bit easier to talk about: notation of derivatives. I have already described a few ways to express these values. I talked about expressing them as limits, and using infinitesimally smaller intervals, and that is a good way to work through them. Symbolically, I said that you can write f'(x) to denote the derivative of the functions f(x). This will likely be the easiest way for you to use it and to recognize it, though here are a few others that mean the same thing:

Each of these terms means the exact same thing. In particular, the D and d/dx are specifically called the differentiation operators, and you can see they have a few variations. Similarly, dy/dx is symbolic of derivatives for historical reasons. Read up on Gottfried Wilhelm Leibniz to learn more about the origins of calculus, where you will see that he introduced this way of representing it. Sometimes, you may see dy/dx referred to as "Leibniz notation."

And with that final tidbit of mathematical goodness, I am going to end this post. I intend to follow this with another post in the near future that introduces differentiation methods and strategies. Much like the exponent rules, there are also several differentiation rules, and I hope to be able to explain them for you as well. If you have made it to this point of my post, thanks for reading, and please be sure to click the Facebook Like button below or at the top, and I'd appreciate a Google +1 as well below if this was helpful!

Saturday, March 30, 2013

The Midsegment Theorem

A while ago, I posted a very popular post that explained how to calculate the midpoint of a line. A lot of people have viewed that page, and so I thought that this somewhat related story might also be equally as interesting for my viewers.

Guillermo, over at "Proofs from the Book," has recently posted an interesting discussion about the Midsegment Theorem, which deals with the line that connects the two midpoints of two sides of a triangle. This concept is useful when doing proofs with triangles. According to Guillermo, the midsegmet (or midline) has these properties:

"(1) the midsegment connecting the midpoints of the two sides of a triangle is parallel to the third side and (2) its length is also half of the third side."

If you learn how to recognize this geometrical identity, it will be very valuable to you when working with geometry or trigonometry questions. The post at that site goes into great detail to explain each step of the proof for each of these two theorems. It is fascinating to see the in-depth steps that go towards demonstrating a mathematical proof, so I recommend this page at http://proofsfromthebook.com/2013/03/25/the-midsegment-theorem/ for anyone interested in learning more about the midsegment theorem.

Thursday, March 14, 2013

It's Pi Day 2013!

Happy Pi Day 2013 everyone!

To most of my regular followers, they know exactly what I'm talking about. To many others though, they may think I'm crazy or talking about some special dessert day and wonder if the pie comes with ice cream or whipped cream. Well, I guess if you're really into it, you could celebrate Pi Day with pie. Why not, right?

Pi Day, of course, comes once a year - on March 14. Numerically, if you write your dates with the month first, this is 3-14. Everyone's favorite math constant! Actually, what I just realized is that we are only two years away from Super Pi Day (my name for it) when the year can also be included. Of course, pi is 3.14159.... But if we round it, March 14, 2016 will 3-14-16. How exciting is that? That will truly be more than enough reason to celebrate with some pie!

Monday, March 4, 2013

The Definitive Guide to Domain and Range

When working with functions and their graphs, one of the most common types of problems that you will encounter will be to identify their domain and range. This isn't necessarily a difficult problem to solve, once you know what domains and ranges actually are! After all, these terms sound like they belong in geography or cartography, or a National Geographic magazine! However, rest assured that these concepts are definitely mathematical, and they are a lot simpler than you may first expect. (Make sure you click the Like button if you like this!)

So then, let's start at the beginning. What does it mean when your teacher asks you to find domain? The domain of a function is simply all of the values of x that a function can have. (I guess you can kind of think of this in terms of a map after all... the domain includes all of the x values where your curve lives.) A very important point to understand with this definition is that it strictly refers to the values of x for your function, no matter the corresponding y values. Consider it as where horizontally your graph sits on the axes. With that in mind, here is a neat way of visualizing this. Since the y values don't matter, pretend that you can take your graph and squash it down so that it is nothing but a horizontal line. Now if you look at this squished version of your curve, you can simply tell what the domain is by where this line sits along the x-axis. This is a very basic way of looking at this concept, but hopefully it does a good job of introducing it.

Moving along to the other half of our topic, now that you know the definition of domain, range must surely be something similar, right? If we were just talking about x values, maybe range deals with y values? Of course it does! The range of a function is all of the values of y that a function can have. Similarly, you can consider this to be where vertically your graph sits on the number lines. Again, you can try to visualize this better by squishing your graph from the left and right into a single vertical line, and then you can tell what y values should be included.

So with those definitions, you should hopefully be able to solve some of your math questions. However, I am going to mention a few other things that you need to consider when working with problems such as these.

The first point you need to assess is whether a graph that you are given is actually a function or not. Not every graph is! Naturally, you may think that now there is going to be some kind of weird calculation or something you have to do to determine this. Well, there is, but it's not nearly as complicated as you think! To decide if a graph is truly a function, it simply has to pass the vertical line test. And yes, that is as easy as it sounds! Take a look at your graph, and place your pencil vertically on it (or draw a bunch of vertical lines through your graph), in the direction of the y-axis. As you move your pencil left and right, does it only ever cross the line at one point at a time, or does it cross more than once? If more than one point on the graph ever touches your vertical pencil at the same time, it is NOT a function. If your vertical lines only pass through the curve at a single point, then it is a function.

What does the vertical line test actually tell you to allow you to have confidence that you are truly working with a function? It demonstrates that for any value of x that you input into the expression, you will only ever get a single value for y. A function always has one y value for each x value. Imagine a straight line: for every x there is a corresponding y. Now, compare to a more advanced curve, such as a circle. A circle would obviously fail a vertical line test, because you can easily see that you get two y values in its range for each x value in its domain (except at the extreme ends). As simple an object as you may think a circle is, it actually is not a function for this very reason.

So that is the first part addressed: determining if your graph is a function in the first place. Having decided that it actually is one, you can then move on to finding the domain and range of a function. This step can actually be remarkably simple, or require a little bit of work. In the simplest case, you will have a continuous curve on a graph (no gaps!) with labeled points, and you can simply read off of the graph what the x values are on the far left and right side of you line, and then state that domain contains all x values between those two points. Equally, you can do the same sort of thing with the y values. Look at the highest and lowest points on the graph, and assuming no holes in the curve, your range is going to be all values contained between those two extreme points.

I should add a very important note here. Not all functions have end points. In fact, unless it is specifically defined in the expression, you may say that most functions do not have end points. Instead, we say that they extend to infinity. As such, it is not uncommon to say that a domain or range is from negative infinity to positive infinity. Consider a line such as f(x) = 3x. You could substitute values into x such as -13848, 4892/38577, or 10000000000, and then calculate the corresponding y. The line extends both left and right, and up and down, forever.

f(x) = 3x

On the other hand, some functions may be defined to purposefully limit their ends. This would be the case when given the expression f(x) = x + 2, for x > 0. In this case, the equation explicitly states that the curve only exists over the positive values for x. So, we would say that its domain ranges from 0 to positive infinity. For the range, in this case, your lowest point is your restricted x value, so you can plug that into the equation to give f(0) = 2. Therefore, your range includes all values from 2 to positive infinity.

f(x) = 2x, x > 0

Now, what about if the equation of the curve is more complicated. What do we do if we have something like f(x) = (2x + 1) / x. It doesn't look that hard. There isn't any rearranging or algebra that needs to be done. You can produce a table of values very simply by subbing in many values for x and determining the y values, and then you can plot the curve. When you do that, you will see that the graph looks more complex that the continuous lines we've seen so far.

f(x) = (2x + 1) / x

You can see that as the curve approaches x = 0, there doesn't appear to actually be point precisely at x = 0. Instead, the curve appears to be asymptotic to the line x = 0, and similarly at the line y = 2. This is a result of the curve being undefined there. This may sound complicated, but it's not at all. A curve is undefined when the denominator of your equation is equal to zero. This is because you cannot divide something by zero. Try it on your calculator. Punch in any number, divide it by zero, and it will return an error. In this case, you can see from the graph that the domain includes all numbers except 0, and that for the range, it appears to include all numbers except y = 2. If you were to only look at the equation, you could also equally say that the domain includes all numbers except zero, because only by putting zero in the denominator would your equation be undefined. To determine the range, you would need to do some rearranging to express it in terms of y. In this case, you would come up with the rearranged formula x = 1 / (y - 2). I'll leave this for you to work through and verify. Going back to our rule that you can't have a zero in the denominator, this curve is undefined when you just say that y - 2 = 0, leading to y = 2. And comparing this to our curve, you can indeed see that this is the case.

I should also discuss graphing conventions and proper notation for expressing domain and range, because there is a connection between the two. If you have a short line segment, say f(x) = 2, extending between and including the points at f(1) and f(5), this curve obviously does not extend to infinity.

f(x) = 2

Because its end point include those points (i.e., 1 < x < 5), you would note this on the graph with a solid dot. (Unfortunately, I haven't figured out this functionality on my graphing program, so take my word for it!) However, if I said that the domain extended between those two points but did not actually include them (i.e. 1 < x < 5), you would represent this with an open dot (like an 'o') at those points. Pay attention to what is happening and this points, because this now affects how you express the domain. You denote domain and range by using either round or square brackets, depending on if you include the point or go right up to the point but don't include it. So, if you have a domain of 1 < x < 5, you can write this as [1, 5]. Similarly, if your domain is 1 < x < 5, you could write (1, 5). Don't get these confused as being ordered pairs! You can also mix and match these brackets, so you can have something like (1, 5] as well. Importantly, if you are stating that your domain or range includes infinity, infinity always gets round brackets. This is because you cannot include the "infinity point" precisely. You are always approaching it.

If you are given a piecewise function, determining the domain and range of that can be a bit trickier. You need to pay attention to all parts of the expression that you are provided, and make a note of anywhere that any of them are undefined. Treat each part individually. Also note the domains and ranges over which each part is defined, and whether there are any gaps in the line.

As you get to higher levels of graphing in algebra and calculus, things will get more complicated. You will have curves that are irregular that require you to analyze their equations. Determining precise points on them will require analysis of their equations, and you won't be able to identify them merely by looking at the curves. You will undoubtedly find problems where you wish you had a domain and range calculator, but all of these problems are solvable if you approach them the right way.

I hope that this post has explained this very common and rudimentary graphing concept in a way that is understandable. It doesn't need to be a complicated as some students think, nor as some teachers make it! Practice with easy examples until you are comfortable enough to move on, and before you know it, you will be an expert at finding the domain and range of a function, and you won't need help with math anymore.

Friday, March 1, 2013

Understanding Point Slope Form

When you are graphing straight lines, one of the most common formats for describing the equation of the line is called "point slope form." In this representation, the equation identifies one ordered pair that is on the line, and the slope. If you were given only those pieces of information, you would have all that you would need to construct the line. Continue reading to learn more about this line graphing concept, and make sure that you Like my post if it is helpful to you!

By using this formula...
- if you know one point that is on the line (x₁, y₁),
- and you know the slope of the line (m),
... with a little bit of mathematics and algebraic rearrangement, you can determine any other point (x, y) on the line.

Here's one type of problem that you will likely encounter: Express in point slope form the line that passes through (4, 2) and has a slope of 8.

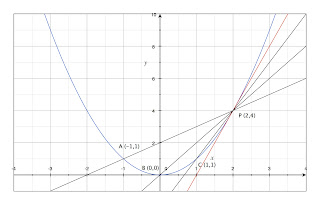
To correctly solve this problem, all that you need to do is substitute the given values into the equation shown above. Therefore, the correct expression is y - 2 = 8(x - 4). It's as easy as that!

A more complicated problem would be something like this: What is the y-coordinate when x = 3 on the line that passes through (1, 1) and has a slope of 5?

To successfully work through this problem, you first approach it as you did the previous one. That is, find the equation of the line. In this case it is y - 1 = 5(x - 1). Now, to find y when x = 3 is as simple as subbing in x = 3 into this equation, doing a bit of rearranging, and simplifying to isolate y. Try it out for yourself, and you will see that y = 11 when x = 3. In other words, the point (3, 11) is on the line that is described in the question.

Now that you've seen a few questions that can be asked about point slope form, perhaps it might help you to better understand the concept if you see what it actually means. First off, consider all of the variables that are included in the expression:

We have an ordered pair (x₁, y₁), an unknown point (x, y) that can be any point on the line at all, and the slope (m). Now, my question to you is: where have you seen these variables together in one place before?

If you answered that this is a rearrangement of the slope formula, then you get a gold star! As we've seen before, the slope of a line is equal to rise over run. In other words, the slope corresponds to the ratio of the change in vertical height to the change in horizontal distance of the line. Mathematically, here is what you this means:

So, really, all we're really dealing with for any of this is the definition of the slope! The point slope formula is just a different way of looking at it.

Now, it should be said that this may not be the most intuitive way of representing an equation of a line. Many people find expressing their equation in the form of y = mx + b to be more familiar and descriptive, since by definition it denotes the slope and y-intercept. The intercept is a very easy "starting point" from which to extend your line, and with the known slope, is it simple to count spaces to plot another point. Whichever way you express the equation of your line, assuming that the math is correct, they are just different ways of describing the same thing and so they aren't wrong... of course, unless your teacher specifically asks for one form or the other.

If you think about it, you should be able to see the connection between "point slope" and "slope intercept" forms. Consider that in y = mx + b, the b term is the y-intercept (or y₁), which means that x₁ = 0. So, you can say that:

y = mx + y₁

y - y₁ = mx

Looks familiar, except it is missing the x₁ term... but we already designated that it is 0 anyways!

Another way to show that these two formulas are the same thing is to equate them to the same variable, which therefore means the equations are equal. This is like solving a system of equations.

If we solve for m in the point slope equation, we have: m = (y - y₁) / (x - x₁).
If we solve for m in the slope intercept equation, we have: m = (y - b) / x.

Since we're talking about the same line, it obviously is the same slope in each version, so it is fair to equate them. So:

(y - y₁) / (x - x₁) = (y - b) / x

Written like this, it is easy to see how the two equations correspond to each other.

A third way of representing a line's equation is to express it in standard form. What this does is put everything on to one side of the expression, simplifies it, and sets it equal to 0. Your expression will then have the form Ax + By + C = 0, where A is the "x" coefficient, B is the "y" coefficient, and C is the constant not associated with either x or y.

The whole point of all of this is to make a very simple point: all you need to fully describe the equation of a straight line is its slope and a point on it. If you only have two points given, it is easy to calculate the slope from the slope formula, and then it is only a matter of plugging numbers into whichever expression you like. If you only have the slope and no points, then you have the shape of a line but no point to which you can anchor it. Get the slope, get a point, pick a way of expressing the equation of your line, and that's all there is to it. If you made it all the way to the end, please remember to click the Like or +1 buttons (or both) below if you enjoyed this post! Thanks!

Wednesday, February 20, 2013

The Largest Known Prime Number

Quick! How many prime numbers can you recite, starting at 2 (1 is not prime!)? Let's see... 2, 3, 5, 7, 11, 13, 17, 19, 23... the further you go, the more you have to think about it, right? What you have to consider is whether each number you evaluate is only divisible by itself and 1, or if it can be divided by other numbers. If it's only 1 and itself, you've found a prime number! But as you count up higher, there is a higher likelihood that these bigger numbers are going to have some out-of-the-ordinary factors that you don't automatically think about. So then, you can see the role that computers play in finding prime numbers.

Computers can do all of the hard calculations. They can check to see for any given number if another number can completely divide into it. This may seem simple if you consider a number like 35 (which isn't prime, because there is 1x35 and also 5x7), but what about considering a number like 64,092,283? It's not likely that you are going to check to see if it has any factors in a short amount of time, but a computer can do it relatively quickly. Of course, the larger the number, the more calculations it has to check and so even they will take some time.

When you get up to the REALLY large numbers, you have to consider that the computer needs lots of time to evaluate all possible factors for each number. Since prime numbers become more rare as you count higher, a larger portion of the values that the computer has to spend time evaluating will actually work out to be non-primes. For this reason, the most recent prime number was discovered FOUR YEARS after the previous prime was reported.

There is currently a distributing computing project named GIMPS (Great Internet Mersenne Prime Search) that is actively doing calculations and checks to find the next prime number. Curtis Cooper from the University of Central Missouri was the one who reportedly found this latest prime, and it is easy to see why it has taken so long to find. This largest prime number ever found has more than 17 MILLION digits. It is the number represented by 2 ^57,885,161 -1. Now, imagine having to check that number by hand to see if it had any other factors to rule it out as a prime. This may not be the most glamorous job for a computer to do, certainly not as entertaining or visually appealing as editing digital photographs or playing video games, but it definitely is a job meant for a computer.

On that note, Phillip Bump got creative with this 17 million digit monster. He divided it into 6 digit chunks, each chunk then representing an RGB colour, and then plotted out the resulting colour distribution. From that point of view, the following picture is essentially the fingerprint of this prime. Maybe working with primes IS a visual art!

The Largest Known Prime Number - by Phillip Bump

Saturday, February 9, 2013

Free Online Courses with Credits - Update

In one of my recently published posts here on my site, I discussed some options available for people looking to find free online courses with certificates. Massive open online courses (MOOCs) have had an increasing role in education discussions over the last couple of years, and world-class institutions such as Harvard and Stanford now offer their own versions of distance learning degrees. In very coincidental timing, I came across an article since I put up that post that further promotes MOOC online classes.

In a move that may go a long ways towards giving online degree courses more recognition and respect, the California State University system has announced a partnership with Udacity, a startup company that produces online college classes, to begin offering a series of introductory courses FOR CREDITS. Subjects offered will include remedial algebra, college level algebra, and introductory statistics. For the time being, these courses are being offered to a limited number of students, for the price of $150, which is many times cheaper than a more typical college or university tuition. These MOOC online classes will involve watching videos and participating in online interactive exercises and quizzes. Students will also have access to online assistance from professors or other mentors.

In a similarly high profile news update, Coursera (arguably the most popular and familiar platform for offering free online education) has announced that it has received approval to make five of its courses available for college transfer credits, including mathematically oriented subjects such as pre-calculus, single variable calculus, and intermediate algebra. Naturally, this can be viewed as an experiment before potentially increasing the number of its courses that would qualify for this credit equivalency.

These massive online courses are gradually becoming more popular and important in schools' education programs and policies, as can be seen by the increasing number of schools that have begun offering variations of these correspondence courses and the availability of transferrable credits towards a degree. One of the main problems, however, is the alarmingly high dropout rate. Some of these MOOC online degree courses have 90% dropouts. That may be because of a high number of people who sign up to just browse the course without ever having an intention of seeing it through to completion, or some may find that there is not enough support or assistance offered, something that regular attendees of campus institutions take for granted. As such, this new program by Udacity will include many mentors available to assist and other student support services, such as check-ins and email encouragements - simple but effective touches to keeping students interested and motivated.

While these programs should probably best be viewed as pilot programs with uncertain futures, if they are received well it should be expected that they will increase their course offerings that carry transfer credits. This will go a long ways to proving the value of the MOOC concept, and will likely cause a cascade of educational institutions to begin offering their own variations of the platforms. It feels as though we may be at a pivotal point in education history. Learning has traditionally been done in schools with lectures, hands-on experience, and supervision - all at high cost - while online education programs - especially the free ones - have typically been regarded as supplementary to these customs. However, with some of these platforms gaining credit equivalency, these may be some of the first steps for these services towards gaining recognition for providing meaningful education that is widely available and affordable - a real education revolution.